Non Singular Matrix Meaning | An invertible matrix has a single solution for every possible $b$ in $ax=b$. Are the following matrices singular? Matrix elements may also be differential operators, … английский словарь британика. A matrix b such that ab = ba = i is called an inverse of a. # this code is contributed by.
If a does not have an inverse, a is called singular. It's easy to imagine that that invertible matrices would be called 'singular'. A matrix is singular if and only if its determinant is zero. There is no multiplicative inverse, b, such that the original matrix a × b = i (identity matrix). This means that a singular matrix is.

Any matrix b with the above property is called an inverse of a. A matrix is singular if and only if its determinant is zero. Definition of nonsingular matrix in the definitions.net dictionary. If a does not have an inverse, a is called singular. $a$ is nonsingular if and only if the column vectors of $a$ are linearly independent. In case the matrix has an inverse, then the matrix multiplied by its. A square matrix whose determinant is not zero. Are the following matrices singular? A matrix b such that ab = ba = i is called an inverse of a. This means that a singular matrix is. # this code is contributed by. If $a$ is nonsingular, then $a^{\trans}$ is nonsingular. From introductory exercise problems to linear algebra exam problems from various universities.
(non singular matrix) an n n a is called non singular or invertible if there exists an n n matrix b such that ab = i n = ba. A singular matrix has a determinant of zero and therefore has no inverse. (inverses are unique) if a has inverses b and c. A matrix is singular if and only if its determinant is zero. Are the following matrices singular?
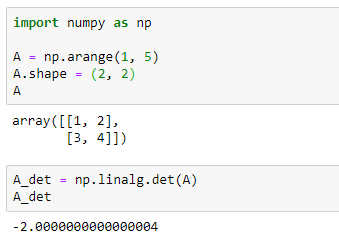
A singular matrix has a determinant of zero and therefore has no inverse. A matrix b such that ab = ba = i is called an inverse of a. Any matrix b with the above property is called an inverse of a. If $a$ is nonsingular, then $a^{\trans}$ is nonsingular. Definition of nonsingular matrix in the definitions.net dictionary. A little noise makes the system go from theoretically impossible to solve to for a singular matrix, the latter is zero: (inverses are unique) if a has inverses b and c. In case the matrix has an inverse, then the matrix multiplied by its. A square matrix whose determinant is not zero. There is no multiplicative inverse, b, such that the original matrix a × b = i (identity matrix). Check out the pronunciation, synonyms and grammar. An invertible matrix has a single solution for every possible $b$ in $ax=b$. Here are all the possible meanings and translations of the word nonsingular matrix.
There can only be one inverse, as theorems 11.4 and 11.5 together imply that a is nonsingular if and only if it is row equivalent to i. I gotta say, this is a very low probability event, sufficiently so that i doubt you can devise a random test to detect any discrepancy. # this code is contributed by. Check out the pronunciation, synonyms and grammar. Definitions for nonsingular matrix non·sin·gu·lar ma·trix.

There is no multiplicative inverse, b, such that the original matrix a × b = i (identity matrix). Presumably to solve some system of equations. If $a$ is nonsingular, then $a^{\trans}$ is nonsingular. Any matrix b with the above property is called an inverse of a. There can only be one inverse, as theorems 11.4 and 11.5 together imply that a is nonsingular if and only if it is row equivalent to i. Definition of nonsingular matrix in the definitions.net dictionary. Tekil olmayan matris, tekil olmayan dizey. A matrix b such that ab = ba = i is called an inverse of a. A matrix is singular if and only if its determinant is zero. Are the following matrices singular? An invertible matrix has a single solution for every possible $b$ in $ax=b$. Any matrix b with the above an important class of non singular matrices is that of the elementary row matrices. In case the matrix has an inverse, then the matrix multiplied by its.
I gotta say, this is a very low probability event, sufficiently so that i doubt you can devise a random test to detect any discrepancy singular matrix meaning. Definition of nonsingular matrix in the definitions.net dictionary.
Non Singular Matrix Meaning: From one of the property of determinants (all elements in the first row are zero which means that its determinant is equal to zero), we know that determinant of.
Fonte: Non Singular Matrix Meaning
0 Response to "Figo! 36+ Fatti su Non Singular Matrix Meaning! A matrix is singular if and only if its determinant is zero."
Posting Komentar